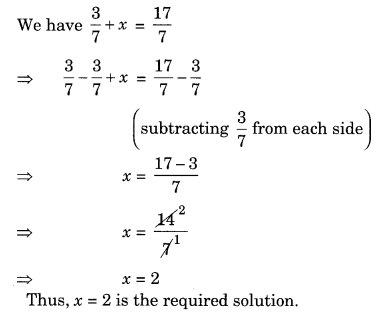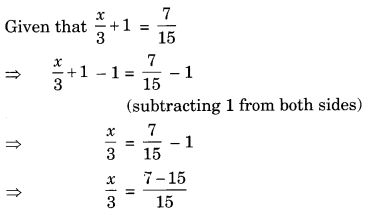Multiplication of algebraic fractions is not so difficult. You need to multiply the numerators, denominators of all fractions together to get its product. Before multiplying the algebraic fractions, factorize them. Check out the simple steps of multiplication of algebraic fractions and solved examples in the following sections.

How to find the Product of Algebraic Fractions?

Here we are giving the simple steps to calculate the multiplication of two or more algebraic fractions. Follow these instructions to get the product quickly.

• Find the factors of numerators, denominators of algebraic fractions.
• If there are any like factors, then cancel them.
• Multiply the numerators of remaining factors and denominators.

Solved Examples on Multiplication of Algebraic Fractions

Example 1.

Simplify 5 / (a + a²) x (a³ – a) / ab?

Solution:

Given that,

5 / (a + a²) x (a³ – a) / ab

Get the factors of both fractions

= 5 / a (a + 1) x a (a² – 1) / ab

= 5 / a (a + 1) x [a (a + 1) (a – 1)] / ab

Multiply the two algebraic fractions.

= 5a (a + 1) (a – 1) / a (a + 1) ab

Cancel the terms a (a +1) in both denominator and numerator.

= 5 (a – 1) / ab

Example 2.

Find the product of the algebraic fraction [5a / 2a-1 – (a-2) / a] x [2a / (a+2) – 1 / (a+2)?

Solution:

Given that,

[5a / 2a-1 – (a-2) / a] x [2a / (a+2) – 1 / (a+2)]

The least common multiple of denominators of the first part is a(2a – 1) and the L.C.M of denominators of the second part is a + 2.

Therefore, [5a. a / a(2a – 1) – (a – 2) . (2a – 1) / a(2a – 1)] x [2a / (a+2) – 1 / (a+2)]

= [(5a² – (a – 2) (2a – 1)) / a(2a – 1)] x [(2a – 1) / (a+2)]

= [(5a² – 2a² + a + 4a – 2) / a(2a – 1)] x [(2a – 1) / (a+2)]

= [(3a² + 5a – 2) / a(2a – 1)] x [(2a – 1) / (a+2)]

= [(3a² + 6a – a – 2) / a(2a – 1)] x [(2a – 1) / (a+2)]

= [(3a (a + 2) -1(a + 2)) / a(2a – 1)] x [(2a – 1) / (a+2)]

= [(a + 2) (3a – 1) / a(2a – 1)] x [(2a – 1) / (a+2)]

= [(a + 2) (3a – 1) (2a – 1)] / [a(2a – 1) (a+2)]

Here the common factors in the numerator and denominator are (a+2), (2a-1). Cancel these factors in both to find the lowest form

= (3a – 1) / a

Therefore, [5a / 2a-1 – (a-2) / a] x [2a / (a+2) – 1 / (a+2)] = (3a – 1) / a.

Example 3.

Find the product and express in the lowest form: 5x² / (x² – 2x) x (x² – 4) / (x² + 2x)?

Solution:

Given that,

5x² / (x² – 2x) x (x² – 4) / (x² + 2x)

Ge the factors of both fractions.

= 5x² / x(x – 2) x (x² – 2²) / (x(x + 2))

Cancel the common term x in the first part.

= 5x / (x – 2) x (x + 2) ( x – 2) / (x(x + 2))

Cancel the common factor (x+2) in the second part.

= 5x / (x – 2) x (x – 2) / x

Multiply both numerators and denominators

= 5x . (x – 2) / x . (x – 2)

Cancel the common factor (x – 2) in both numerator and denominator.

= 5x/ x

= 5.

∴ 5x² / (x² – 2x) x (x² – 4) / (x² + 2x) = 5.

Example 4.

Find the product of the algebraic fractions in the lowest form:

[(x + 2y) / (2x + y)] x [(2x + 5y) / (x + y)]

Solution:

Given that,

[(x + 2y) / (2x + y)] x [(2x + 5y) / (x + y)]

= [(x + 2y) (2x + 5y)] / [(2x + y) (x + y)]

= [2x² + 5xy + 4xy + 10y²] / [2x² + xy + 2xy + y²]

= [2x² + 9xy + 10y²] / [2x² + 3xy + y²]

Example 5.

Simplify (4x² – 1) / (9x – 6) x (15x – 10) / (x + 4)?

Solution:

Given that,

(4x² – 1) / (9x – 6) x (15x – 10) / (x + 4)

Calculate the factors.

= ((2x)² – 1²) / 3(3x – 2) x 5(3x – 2) / (x + 4)

= (2x + 1) (2x – 1) / 3(3x – 2) x 5(3x – 2) / (x + 4)

Multiply numerators, denominators together.

= [(2x + 1) (2x – 1) . 5(3x – 2)] / [3(3x – 2) (x + 4)]

Cancel the terms (3x – 2)

= [5(2x + 1) (2x – 1)] / 3(x + 4)

∴ (4x² – 1) / (9x – 6) x (15x – 10) / (x + 4) = [5(2x + 1) (2x – 1)] / 3(x + 4).

The post Multiplication of Algebraic Fractions | How to Multiply Algebraic Fractions? appeared first on Learn CBSE.

The division is one of the arithmetic operations. The division of algebraic fractions is similar to the division of numbers. Here, you need to factorize the numerators and denominators of the fractions. Cancel the like factors in a fraction and reverse the denominator fraction and multiply it with numerator fraction to get the division value. Find the solved example questions on the division of algebraic fractions.

How to Divide Algebraic Fractions?

Check the simple and easy steps to divide algebraic fractions in the following sections.

• Take two algebraic fractions. One in the numerator and the second in the denominator.
• Find the factors of fractions.
• Get first fraction x 1 / second fraction.
• Cancel the like terms in the numerator and denominator.
• Multiply the numerators, denominators to get the result.

Solved Examples on Dividing Algebraic Fractions

Example 1.

Determine the quotient of the algebraic fractions: x / (x² – x) ÷ 10x / (x² + x – 2)?

Solution:

Given that,

x / (x² – x) ÷ 10x / (x² + x – 2)

Factorize the fractions and cancel the common terms.

x / x (x – 1) ÷ 10x / (x² + 2x – x – 2)

= x / x (x – 1) ÷ 10x / (x(x + 2) -1(x + 2))

= x / x (x – 1) ÷ 10x / (x + 2) (x – 1)

= 1/(x – 1) ÷ 10x / (x + 2) (x – 1)

= 1/(x – 1) x (x + 2) (x – 1) / 10x

= (x + 2) (x – 1) / 10x (x – 1)

= (x + 2) / 10x

Example 2.

Divide the algebraic fractions and express in the lowest form: 9x²+12x+4 / 4x²-27x-7 ÷ 12x²+5x-2 / 16x²-1?

Solution:

Given that,

9x²+12x+4 / 4x²-27x-7 ÷ 12x²+5x-2 / 16x²-1

Factorize the fractions and cancel the common terms.

= 9x²+6x+6x+4 / 4x²-28x+x-7 ÷12x²+8x-3x-2 / (4x)²-1²

= 3x(3x+2)+2(3x+2) / 4x(x-7)+1(x-7) ÷ 4x(3x+2)-1(3x+2) / (4x+1)(4x-1)

= (3x+2)(3x+2) / (x-7)(4x+1) ÷ (3x+2)(4x-1) / (4x+1)(4x-1)

= (3x+2)(3x+2) / (x-7)(4x+1) ÷ (3x+2) / (4x+1)

= (3x+2)(3x+2) / (x-7)(4x+1) * (4x+1) / (3x+2)

= (3x+2)(3x+2)(4x+1) / (x-7)(4x+1)(3x+2)

= 3x+2 / x-7

Example 3.

Find the quotient of the algebraic fractions:

x²+11x+24 / x²-15x+56 ÷ x²-x-12 / x²-11x+28

Solution:

Given that,

x²+11x+24 / x²-15x+56 ÷ x²-x-12 / x²-11x+28

Factorize the algebraic fractions and cancel the common terms.

= x²+8x+3x+24 / x²-8x-7x+56 ÷ x²-4x+3x-12 / x²-7x-4x+28

= x(x+8)+3(x+8) / x(x-8)-7(x-8) ÷ x(x-4)+3(x-4) / x(x-7)-4(x-7)

= (x+8)(x+3) / (x-8)(x-7) ÷ (x-4)(x+3) / (x-4)(x-7)

= (x+8)(x+3) / (x-8)(x-7) ÷ (x+3) / (x-4)

= (x+8)(x+3) / (x-8)(x-7) * (x-4) / (x+3)

= (x+8)(x+3)(x-4) / (x-8)(x-7)(x+3)

= (x+8)(x-4) / (x-8)(x-7)

= x²+4x-32 / x²-15x+56

The post Division of Algebraic Fractions | How to Divide Algebraic Fractions? appeared first on Learn CBSE.

Learn How to Change the Subject of a Formula and also know about finding the value of the variables by reading the complete article. We included solved examples with clear explanations for better understanding. Students can immediately practice all the questions available in this article and learn the best ways to Change the Subject of a Formula.

Subject of the Formula

Expressing one variable in terms of other variables is the main concept of the formula. The variable that expresses in other variables is called the subject of the formula. The subject of the formula will be written on the left side and other constants and variables are written on the right side of the equality sign in a formula.

Example:
z = xy, where z is the subject of the formula where it is expressed in terms of the product of the x and y.
If we want to change the subject of the formula to x, then the above expression will change into x = z/y.

How to Change the Subject of the Formula?

Changing the subject of a formula can be possible by rearranging the formula to get the required subject. To change the subject of the formula, firstly change its side and change the operation.

When one variable moved to the other side of the equal to sign, the operation becomes inverse. For example, if a variable is added to the subject of the formula, then it will be subtracted after moving to the other side of the equal to sign.

Examples:

1. Make ‘v’ the subject of the formula in u = v + as

Solution:
Given that u = v + as
as is added to v.
To find the subject of the v subtract the as from both sides.
u – as = v + as – as
u – as = v

The final answer is v = u – as

2. Make ‘t’ the subject of the formula, s = x + bt

Solution:
Given that s = x + bt
x is added to the bt.
Firstly, subtract x on both sides.
s – x = x – x + bt
s – x = bt
b is multiplied to t.
Divide b on both sides.
(s – x)/b = bt/b
(s – x)/b = t

The final answer is t = (s – x)/b.

Change the Subject of a Formula Solved Examples

1. The volume of a box is the product of the length and breadth of the box?

Solution:
Given that the volume of a box is the product of the length and breadth of the box.
The volume of a box = v
The length of the box = l
The breadth of the box = b
v = l × b
If the subject of the box is length, the answer is l = v/b
If the subject of the box is the breadth, the answer is b = v/l.

The answer is v = l × b, l = v/b, and b = v/l.

2. In the relation x/5 = make (s – 16)/7 make s as the subject.

Solution:
Given that In the relation x/5 = make (s – 16)/7 make s as the subject.
x/5 = (s – 16)/7
7 is dividing (s – 16)
Multiply 7 on both sides.
7x/5 = (s – 16)7/7
7x/5 = (s – 16)
16 is subtracted from the s.
Add 16 on both sides of the equation.
7x/5 + 16 = s – 16 + 16
7x/5 + 16 = s

The final answer is s = 7x/5 + 16.

3. Make t the subject of the formula s = (t + r)/(t – r)

Solution:
Given that s = (t + r)/(t – r)
Multiply (t – r) on both sided.
s(t – r) = (t + r)
st – sr = t + r
To find the t as subject of the formula, move t variables on left side.
Subtract t on both sides.
st – t – sr = t – t + r
st – t – sr = r
Take t as common from st – t
t (s – 1) – sr = r
Add sr on both sides
t(s – 1) – sr + sr = r + sr
t(s – 1) = r(1 + s)
Divide (s – 1) on both sides
t(s – 1)/(s – 1) = r(1 + s)/(s – 1)
t = r(1 + s)/(s – 1)

The final answer is t = r(1 + s)/(s – 1).

4. Write the formula for finding the area of the rectangle and indicate the subject in this formula. Also, make b as the subject. If A = 24 cm² and l = 4 cm, then find b.

Solution:
The area of the rectangle is A = l × b.
To make the b as the subject of the formula, divide l on both sides.
A/l = lb/l
A/l = b
b = A/l
Now, substitute the values of l and A.
b = 24/4
b = 6.

Therefore, b = 6 is the answer.

5. For a right angled triangle abc, square of the hypotenuse (h) is equal to the sum of squares of its other two sides (s, t).
• Frame the formula for the above statement and find out h if s = 3 and t = 2.
• Also, make ‘s’ the subject of the formula and find s if h = 8 and t = 6.

Solution:
From the given data, Frame the formula for the above statement and find out h if s = 3 and t = 2.
The formula is h² = s² + t²
Substitute s = 3 and t = 2 in h² = s² + t²
h² = 3² + 2² = 9 + 4 = 13
h = √13

The answer is h = √13

From the given data, make ‘s’ the subject of the formula and find s if h = 8 and t = 6.
By changing the subject to s, the h² = s² + t² becomes s² = h² – t²
Substitute h = 8 and t = 6 in s² = h² – t²
s² = 8² – 6²
s² = 64 – 36
s² = 28
s = √28

The answer is s = √28.

6. In the formula, t = s + (b – 1)d make d as the subject. Find d when t = 10, s = 2, b = 5.

Solution:
Given that t = s + (b – 1)d.
Subtract s on both sides
t – s = s – s + (b – 1)d.
t – s = (b – 1)d.
Divide (b – 1) on both sides
(t – s)/(b – 1) = d
d = (t – s)/(b – 1)
Substitute t = 10, s = 2, b = 5.
d = (10 – 2)/(5 – 1) = 8/4 = 2
d = 2.

The final answer is d = 2.

The post Change the Subject of a Formula | How to Change the Subject of a Formula? appeared first on Learn CBSE.

Are you searching for the Changing the Subject in an Equation or Formula Question and Answers? Then, you are at the correct place and you can get them here. All the Problems on Changing the Subject in an Equation or Formula are explained with detailed explanations in this article. Have a look at every problem and completely understand the Changing the Subject in an Equation or Formula concept.

Procedure for Changing Subject in an Equation or Formula?

Changing the Subject in an Equation or Formula is the way of changing the subject of formula and method of substitution to find the value of one variable. The method of substitution is the process of substituting the given values in the place of variables to find the value of the algebraic expression.

• Find the required variable as the subject.
• Note down the given values and substitutes them in the concern variables.
• Finally, simplify the expressions and find the value of the subject.

Solved Examples on Changing the Subject in an Equation or Formula

1. Make s the subject of the below Adding and Subtracting formula
(i) t = s + r
(ii) t = s – r

Solution:
(i) Given that t = s + r
Subtract r on both sides
t – r = s+r-r

t-r = s
The final answer is s = t – r
(ii) Given that t = s – r
Add r on both sides
t + r = s-r+r
The final answer is s = t +r

2. (i) Given x = ty Make y as the subject.
(ii) Given p = rq Make r as the subject.

Solution:
(i)Given that x = ty
t is multiplying y.
Divide t on both sides to get y as the subject.
x/t = y

The final answer is y = x/t

(ii) Given that p = rq
q is multiplying r.
Divide q on both sides to get r as the subject.
p/q = r

The final answer is r = p/q

3. Given B = Q {1 + (t/100)}ⁿ make t as the subject.
Given B = 1102.50 Q = 1000 n = 2, find t.

Solution:
Given that B = Q {1 + (t/100)}ⁿ
Divide Q on both sides
B/Q = Q/Q {1 + (t/100)}ⁿ
B/Q = {1 + (t/100)}ⁿ
Apply the power 1/n on both sides
(B/Q)^1/n = {1 + (t/100)}
Subtract 1 on both sides
(B/Q)^1/n – 1 = 1 – 1 + (t/100)
(B/Q)^1/n – 1 = (t/100)
Multiply 100 on both sides
100{(B/Q)^1/n – 1} = t
The answer is t = 100{(B/Q)^1/n – 1}
Substitute the given values B = 1102.50 Q = 1000 n = 2, to find t.
t = 100{(1102.50/1000)^1/2 – 1}
t = 100{(441/400)^1/2 – 1}
t = 100 [{(21/20)²}^1/2 – 1]
t = 100 [(21/20) – 1]
t = 100/20
t = 5

The final answer is t = 5.

4. Make L the subject of the following formula:
(i) s = mL + r
(ii) r = mnL + t

Solution:
(i) Given that s = mL + r
Sutract r on both sides
s – r = mL
Divide m on both sides
(s – r)/m = L

The final answer is L = (s – r)/m

(ii) Given that r = mnL + t
Sutract t on both sides
r – t = mnL
Divide mn on both sides
(r – t)/mn = L

The final answer is (r – t)/mn.

The post Changing the Subject in an Equation or Formula | How to Change the Subject in an Equation or Formula? appeared first on Learn CBSE.

Compound Interest is the Interest calculated on the principal and accumulated interest on the previous period’s loan. Get to know the formula and steps to calculate Compound Interest from here. Check out the Solved Problems on finding the Compound Interest. Try Practicing the Examples over here and refer to them as a quick guide to solve problems on Compound Interest.

Compound Interest Questions and Answers

1. Find the amount if Rs. 10,000 is invested at 10% p.a. for 2 years when compounded annually?

Solution:

We know A = P(1+R/100)ⁿ

From given data P = 10,000

R = 10%

n = 2 years

Substituting the input values we have the equation as under

A = 10,000(1+10/100)²

= 10,000(1+0.1)²

= 10,000(1.1)²

= 10,000(1.21)

= Rs. 12,100

2. Find the CI, if Rs 5000 was invested for 2 years at 10% p.a. compounded half-yearly?

Solution:

We know A = P(1+R/100)ⁿ

From given data P = 5,000

R = 10%

n = 2 years

Substituting the input values we have the equation as under

A = 5000(1+10/100)²

= 5000(1+0.1)²

=5000(1.1)²

= 5000(1.21)

= Rs. 6050

CI = A – P

= 6050 – 5000

= Rs. 1050

3. The CI on a sum of Rs 1000 in 2 years is Rs 440. Find the rate of interest?

Solution:

Given P = 1000

n = 2 years

CI = 150

We know CI = A – P

440 = A – 1000

A = 1440

R = ?

We know the formula for Amount A = P(1+R/100)ⁿ

Substitute the given values in the above formula

1440 = 1000(1+R/100)²

1440/1000 = (1+R/100)²

12/10 = 1+R/100

12/10 -1 = R/100

(12-10)/10 = R/100

2/10 = R/100

Rearranging we get the Rate of Interest as 20%.

4. The difference between SI and CI for 2 years at 10% per annum is Rs 15. What is the principal?

Solution:

We know the formula Difference =  P (R/100)²

15 = P(10/100)²

15 = P(100/10000)

15 = P/100

Therefore, Principal = Rs 1500

5. A certain sum amounts to $ 7200 in 2 years at 6% per annum compound interest, compounded annually. Find the sum?

Solution:

Given Data A = $7200

n = 2 years

R = 6%

Formula to Calculate the Amount A = P(1+R/100)ⁿ

7200 = P(1+6/100)²

7200 = P(106/100)²

7200 = P(1.1236)

P = 7200/1.1236

= $ 6407

Therefore, the Sum is $6407.

6. A man deposited $100000 in a bank. In return, he got $133100. Bank gave interest 10% per annum. How long did he kept the money in the bank?

Solution:

Principal = $100000

A = $133100

R = 10%

n = ?

Formula to Calculate the Amount is A = P(1+R/100)ⁿ

Substitute the input values in the above formula and rearrange it to obtain the value of n

133100 = 100000(1+10/100)ⁿ

133100/100000 = (1+10/100)ⁿ

(11/10)³ = (110/100)ⁿ

(11/10)³ = (11/10)ⁿ

n= 3

Therefore, the man kept his money in the bank for 3 years.

The post Problems on Compound Interest | Compound Interest Problems with Solutions appeared first on Learn CBSE.

Let us discuss how to find the Compound Interest when a Variable Rate is given. Check out the Solved Examples on finding the Compound Interest When Rate of Successive Years is Different. We tried explaining each and every step for all the Problems provided here. Use the Problems over here and learn the concept behind them in no time. After going through this article, you will learn the concept of Variable Rate of Compound Interest quite easily.

How to find Compound Interest When Successive Years Rate of Interest is Different?

Get to know in detail how to find the Compound Interest when Consecutive/ Successive Years Rate of Interest is Different from the below sections.

Let us consider the amount be A and Principal be P,

Rate of Compound Interest for Successive Years is different i.e. r₁%, r₂%, r₃%, r₄%, …… then the Formula to calculate amount is given by

A = P(1+r₁/100)(1+r₂/100)(1+r₃/100)(1+r₄/100)……

Where A = Amount

P = Principal

r₁%, r₂%, r₃%, r₄%, ……  are the rate of successive years

Solved Problems on Variable Rate of Compound Interest

1. Find the compound interest accrued by Amar from a bank on $ 12000 in 3 years, when the rates of interest for successive years are 8%, 10%, and 12% respectively?

Solution:

Formula for Amount A = P(1+r₁/100)(1+r₂/100)(1+r₃/100)(1+r₄/100)……

From given data P = $12,000

n = 3 years

r₁ = 8% r₂= 10% r₃ = 12%

A = 12,000(1+8/100)(1+10/100)(1+12/100)

= 12,000(1+0.08)(1+0.1)(1+0.12)

= 12,000(1.08)(1.1)(1.12)

= $15966

CI = A – P

= 15966 – 12000

= $3966

2. A company offers the following growing rates of compound interest annually to the investors on successive years of investment 5%, 6% and 7%

(i) A man invests $ 30,000 for 2 years. What amount will he receive after 2 years?

(ii) A man invests $ 20,000 for 3 years. What amount he will receive after 3 years?

Solution:

Formula to Calculate the Amount is A = P(1+r₁/100)(1+r₂/100)(1+r₃/100)(1+r₄/100)……

(i) Principal = $30,000

n = 2 years

r₁ = 5%, r₂ = 6%

Substitute the input values in the formula we have the equation as under

A = 30,000(1+5/100)(1+6/100)

= 30,000(1.05)(1.06)

= $ 33,390

Therefore, the Man receives $33,390 by the end of 2 years.

(ii) From the given data

Principal = $20,000

n = 3 years

r₁ = 5%, r₂ = 6%, r₃ = 7%

Substitute the input values in the formula we have the equation as under

A = 20,000(1+5/100) (1+6/100)(1+7/100)

= 20,000(1.05)(1.06)(1.07)

= $23,818

Therefore, the Man receives $23,818 by the end of 3 years.

The post Variable Rate of Compound Interest | Compound Interest Formula with Successive Rate of Interest appeared first on Learn CBSE.

The Major Difference Between Simple Interest and Compound Interest is just that Simple Interest is calculated on the Principal whereas Compound Interest is calculated on the Principal Amount along with the Interest accumulated for a certain period of time. Both Simple and Compound Interest are widely used concepts in the majority of financial services. Check out Solved Example Problems for finding the difference between CI and SI in the later sections. Get to know about the concept Difference of Compound Interest and Simple Interest in detail by going through the entire article.

How to find the Difference Between Simple Interest and Compound Interest?

Let us discuss in detail how to find the Difference between Simple Interest and Compound Interest. They are along the lines

Consider the Rate of Interest is the same for both Compound Interest and Simple Interest

Difference = Compound Interest for 2 years – Simple Interest for 2 Years

= P{(1+r/100)²-1} – P*r*2/100

= P*r/100*r/100

=((P*r/100)*r*1)/100

Solved Examples on Difference of Compound Interest and Simple Interest

1. Find the difference of the compound interest and simple interest on $ 10,000 at the same interest rate of 10 % per annum for 2 years?

Solution:

Simple Interest = PTR/100

= 10,000*10*2/100

= $2000

To find the Compound Interest firstly calculate the Amount

Amount A = P(1+R/100)ⁿ

A = 10,000(1+10/100)²

= 10,000(110/100)²

= 10,000(1.21)

= $12,100

Compound Interest = Amount – Principal

= 12,100 – 10,000

= $2,100

Difference between CI and SI = CI for 2 Years – SI for 2 Years

= $2100- $2000

= $100

2. What is the sum of money on which the difference between simple and compound interest in 2 years is $ 100 at the interest rate of 5% per annum?

Solution:

Simple Interest = PTR/100

Principal = P

T = 2 years

Substituting the given data in the formula for the simple interest we have

SI = (P*2*5)/100

To find the Compound Interest firstly, find out the Amount

Amount A = P(1+R/100)ⁿ

= P(1+5/100)²

CI = Amount – Principal

= P(1+5/100)² – P

= P((1+5/100)² -1)

Given the difference between CI and SI = $100

P((1+5/100)² -1) – (P*2*5)/100 = $100

P((105/100)² -1)-10P/100 = $100

P(1.1025-1)-10P/100 = $100

100P(0.1025)-10P =$10000

110.25P-10P = $10000

100.25P = $10000

P = $10000/100.25

= $99.75

Therefore, the Sum of Money is $99.75

The post Difference of Compound Interest and Simple Interest | How to Calculate Difference Between SI and CI? appeared first on Learn CBSE.

Students can find several questions on Compound Interest. Practice the Objective Questions of Compound Interest Over here and be prepared for the exams. Learn how to solve Compound Interest Problems by checking the Solved Examples. Use the Sample Problems over here covering various questions including the Compound Interest Formula.

Compound Interest Practice Test has questions when the Interest Rate is Compounded Annually, Half-Yearly, Quarterly, Various Rate of Interest, Amount Calculations, etc. Solve the Questions on CI and test your knowledge on the related areas and bridge the gap accordingly.

1. The compound interest on $ 20,000 at 5 % per annum for 3 years, compounded annually is?

Solution:

P = $20,000

R = 5%

n = 3 Years

A = P(1+R/100)ⁿ

= 20,000(1+5/100)³

=20,000(105/100)³

= 20,000(1.157)

= $23152

CI = A – P

= $23152 – $20,000

= $3152

2. The simple interest on a sum of money for 2 years at 3 % per annum is $ 6250. What will be the compound interest on the same sum at the same rate for the same period, compounded annually?

Solution:

From given data SI = $6250

T = 2 Years

R = 3%

SI = PTR/100

6250 = P*2*3/100

6250 = 6P/100

6250*100 = 6P

6P = 625000

P = $1,04,166

We know A = P(1+R/100)ⁿ

=1,04,166(1+3/100)²

=1,04,166(103/100)²

=1,10,509

Compound Interest = Amount – Principal

= $1,10,509 – $1,04,166

= $6343

3. If a sum of Rs. 10,000 lent for 10% per annum at compound interest then the sum of the amount will be Rs. 14,161 in

Solution:

We know A = P(1+R/100)ⁿ

Given P = Rs. 10,000

R = 10%

A = 14,161

n = ?

Substitute the input values in the formula of Amount we have

14161 = 10000(1+10/100)ⁿ

14161/10000 = (1+10/100)ⁿ

(11/10)⁴ = (11/10)ⁿ

n = 4 Years

4. The population of a city is 1,20,000. It increases by 5% in the first year and increases by 10% in the second year. What is the population of the town at the end of 2 yrs?

Solution:

The population of city = 1,20,000

The population of city after 2 years = P(1+R₁/100)(1+R₂/100)

= 1,20,000(1+5/100)(1+10/100)

= 1,20,000(1.05)(1.1)

= 1,38,600

Therefore, the Population of the city by the end of 2 years is 1,38,600.

5. The difference between simple interest and compound on Rs. 1500 for one year at 20% per annum reckoned half-yearly is

Solution:

Given Data is Principal = 1500

R = 20%

T = 1 year

SI = PTR/100

= (1500*1*20)/100

= Rs. 300

Amount A = P(1+R/100)ⁿ

=1500(1+(10/100))²

= 1500(1+1/10)²

=1500(1.1)²

= Rs. 1815

CI = A – P

= Rs. 1815 – Rs. 1500

= Rs. 315

Difference = CI – SI

= Rs. (315 – 300)

= Rs. 15

The post Practice Test on Compound Interest | Compound Interest Questions and Answers appeared first on Learn CBSE.

In our Day-Day Lives, we see entities such as Population of a City, Value of a Property, Weight of a Child, Height of a Tree that grow over a period of time. We call the Increase in Quantity as Growth and the Growth Per Unit Time is known as the Rate of Growth. If the Growth Rate occurs at the Same Rate then we call it Uniform Increase or Uniform Growth Rate. Check the Formulas for Uniform Rate of Growth and the Solved Problems for finding the Principal of Compound Interest in Uniform Rate of Growth explained step by step.

How to find the Uniform Rate of Growth?

Learn how to calculate the uniform growth rate by referring to the below modules.

If the Present Value P of a quantity increases at the rate of r % per unit of time then the value Q of the quantity after n units of time is as such

Q = P(1+r/100)ⁿ

Growth is obtained by subtracting the Increased Value from the Actual Value

Growth = Q – P

= P(1+r/100)ⁿ – P

= P{(1+r/100)ⁿ -1}

Solved Examples on Principal of Compound Interest in Uniform Rate of Growth

1. The population of the town increases by 8% every year. If the present population is 7000, what will be the population of the town after 2 years?

Solution:

Given Current Population = 7000

n = 2 Years

Rate of Interest = 8%

Q = P(1+r/100)ⁿ

Substitute the input values in the above formula

= 7000(1+8/100)²

= 7000(1+0.08)²

= 7000(1.08)²

= 7000(1.1664)

= 8164

Population of a Town after 2 years is 8164.

2. John buys a plot of land for $ 20,000. If the value of the land appreciates by 10% every year then find the profit that John will make by selling the plot after 3 years?

Solution:

P = $20,000

interest rate = 10%

n = 3 years

Q = P(1+r/100)ⁿ

= 20,000(1+10/100)³

= 20,000(1+0.1)³

= 20,000(1.1)³

= $26620

Profit made by John = $26620 – $20,000

= $6,620

3. Mike purchased a bike for Rs. 45,000. If the cost of his bike is appreciated at a rate of 5% per annum, then find the cost of the bike after 3 years?

Solution:

Initial Price = Rs. 45, 000

Rate of Appreciation = 5 % Per Annum

n = 3 years

Q = P(1+r/100)ⁿ

= 45,000(1+5/100)³

= 45,000(1+0.05)³

= 45,000(1.05)³

= Rs. 52093

Therefore, the Cost of the Bike after 3 years is Rs. 52093.

The post Uniform Rate of Growth | How to Calculate Uniform Growth Rate? appeared first on Learn CBSE.

Get to know about the Uniform Rate of Depreciation along with its Formula and Solved Examples. In this article, we will discuss how to apply the Principal of Compound Interest on Problems of Uniform Rate of Depreciation. If the Rate of Decrease is Uniform then we call it a Uniform Decrease or Depreciation. Refer to the Solved Examples on How to find Rate of Depreciation or Decrease. We have listed the Step by Step Solutions for each and every problem making it easy for you to understand.

How to Calculate the Rate of Uniform Decrease or Depreciation?

Let us discuss in detail how to find the Uniform Rate of Decrease or Depreciation in the coming sections.

If the Present Value P of a Quantity Decreases at the rate of r % per unit of time then Value Q of the Quantity after n units of time is given by

Q = P(1-r/100)ⁿ

Depreciation in Value = P – Q

= P – P(1-r/100)ⁿ

= P{1-(1-r/100)ⁿ}

Efficiency of a Machine after regular use, Decrease in the Value of Furniture, Buildings, Decrease in the Number of Diseases, etc. come under Uniform Rate of Decrease or Depreciation.

Solved Examples on Uniform Rate of Depreciation

1. The value of a residential flat constructed at a cost of Rs.1,20,000 is depreciating at the rate of 5% per annum. What will be its value 4 years after construction?

Solution:

From the Given Data P = Rs. 1,20,000

r = 5%

n = 4 Years

We know the formula to find Q = P(1-r/100)ⁿ

Substitute the input values in the above formula and we have the equation as such

Q = 1,20,000(1-5/100)⁴

= 1,20,000(95/100)⁴

= 1,20,000(0.8145)

= Rs. 97,740

Therefore, the Value of a Residential Flat after 4 Years would be Rs. 97,740.

2. The price of a motor vehicle depreciates by 10% every year. By what percent will the price of the car reduce after 2 years?

Solution:

From the given data

Consider the Price be P

r = 10%

n = 2 Years

We know the formula to find Q = P(1-r/100)ⁿ

Substitute the input values in the above formula and we have the equation as such

Q = P(1-10/100)²

=P(90/100)²

= P(9/10)(9/10)

= 81P/100

Reduction in Price = P -81P/100

= 19P/100

Percent Reduction in Price = (19P/100)/P*100%

= 19%

3. The cost of a school bus depreciates by 8 % every year. If its present worth is $ 27,000. What will be its value after three years?

Solution:

From the given data

P = $27,000

r = 8%

n = 3 years

Formula to Calculate the Price of Depreciated Value Q = P(1-r/100)ⁿ

= 27,000(1-8/100)³

= 27,000(92/100)³

= 27,000(0.92)³

= 27,000(0.778)

= $ 21, 024

The Cost of a School Bus after the Depreciation is $ 21,024.

The post Uniform Rate of Depreciation | How to find Rate of Depreciation? appeared first on Learn CBSE.

Practice the Questions based on the Uniform Rate of Growth and Depreciation from here. Learn about the Concept of Uniform Rate of Growth and Depreciation better by going through this entire article. You will find How to Apply Principal of Compound Interest on Combination of Uniform Rate of Growth and Depreciation. Check out Formula, Solved Examples on the concept of Uniform Rate of Increase or Decrease from this article. Get Step by Step Solutions for all the Problems provided and get a good hold on the concept.

How to find the Uniform Rate of Increase or Decrease?

Let us discuss how to find the Uniform Rate of Growth or Depreciation in detail in the below modules.

If a quantity P grows at the rate of r₁% in the first year and depreciates at r₂% in the second year and grows at r₃% in the third year then the quantity becomes Q after 3 years.

Take r/100 with a positive sign for each growth or appreciation of r % and r/100 with a negative sign for depreciation of r%.

Solved Examples on Uniform Rate of Growth or Depreciation

1. The current population of a town is 60,000. The population increases by 10 percent in the first year and decreases by 5% in the second year. Find the population after 2 years?

Solution:

Initial Population = 60,000

r₁ =10%, r₂ = 5%

Population after 2 Years Q = P(1+r₁/100)(1-r₂/100)

= 60,000(1+10/100)(1-5/100)

= 60,000(1+0.1)(1-0.05)

= 60,000(1.1)(0.95)

= 62,700

Therefore, the Population after 2 Years is 62,700.

2. The count of a certain breed of bacteria was found to increase at the rate of 4% per hour and then decrease by 2% per hour. Find the bacteria at the end of 2 hours if the count was initially 2,00,000.

Solution:

Since the Population of bacteria increases and decreases we use the formula

Q = P(1+r₁/100)(1-r₂/100)

= 2,00,000(1+4/100)(1-2/100)

= 2,00,000(1+0.04)(1-0.02)

= 2,00,000(1.04)(0.98)

= 2,03,840

Bacteria at the end of 2 hours is 2,03,840

3. The price of a car is $ 2,50,000. The value of the car depreciates by 10% at the end of the first year and after that, it depreciates by 15%. What will be the value of the car after 2 years?

Solution:

Initial Price of the Car = $ 2,50,000

r₁  = 10% r₂ = 15%

Since the Price of a Car depreciates we use the formula

Q = P(1-r₁/100)(1-r₂/100)

= 2,50,000(1-10/100)(1-15/100)

= 2,50,000(90/100)(85/100)

= 2,50,000(0.9)(0.85)

= $1,91,250

Value of Car after 2 Years is $1,91,250.

The post Uniform Rate of Growth and Depreciation | How to find Uniform Rate of Increase or Decrease? appeared first on Learn CBSE.

Probability is a branch of mathematics that deals with the occurrence of random events. It is expressed from zero to one and predicts how likely events are to happen. In general, Probability is basically the extent to which something is likely to happen. You will learn about Probability Distribution where you will learn the possibility of outcomes for a random experiment.

Probability Definition

Probability is the measure of the likelihood of an event to occur.  In the case of events, we can’t predict with total certainty. We can only predict the cancer of an event to occur i.e. how likely it is to happen. Probability ranges between 0 to 1 in which 0 indicates the event to be an impossible one and 1 indicates a certain event.

Example: For instance, when we toss a coin there are only two possibilities either head or tail(H,T). If we toss two coins in the air there are three possible outcomes that are both the coins show heads, both the coins show tails, one is head and the other is tail i.e. (H, H), (T, T), (H, T).

Formula for Probability

Probability is defined as the possibility of an event to occur. The formula for Probability is given as the ratio of the number of favorable events to the total number of possible outcomes.

Probability of an event to happen = No. of Favourable Outcomes/ Total Number of Outcomes

This is the basic formula for Probability.

Probability Tree

Tree Diagram helps to organize and visualize different possible outcomes. Branches and ends are the two main positions of the tree. Each branch Probability is written on the branch and the ends contain the final outcome. Tree Diagram helps you to figure out when to multiply and add.

Types of Probability

There are three types of major probabilities. They are

• Theoretical Probability
• Experimental Probability
• Axiomatic Probability

Theoretical Probability: It depends on the possible chances of something to happen. Theoretical Probability mainly depends on the reasoning behind probability.

Experimental Probability: This kind of Probability depends on the observation of the experiment. Experimental Probability can be calculated on the number of possible outcomes to the total number of trials.

Axiomatic Probability: A Set of Rules or Axioms are Set applies to all types. Using the axiomatic approach to probability, the chances of occurrence or non-occurrence of the events can be quantified.

Conditional Probability is nothing but the likelihood of an event or outcome occurring based on the occurrence of a previous event or outcome.

Probability of an Event

Let us consider an Event E that occurs in r ways out of n possible ways. The probability of happening an event or its success is given by

P(E) = r/n

The probability of an event or its failure is given by

P(E’) = (n-r)/n = 1-(r/n)

E’ represents the event will not occur.

Therefore, we can say that

P(E)+P(E’) = 1

What are Equally Likely Events?

If the events have the same theoretical probability of happening then they are called Equally Likely Events. Results of Sample Space are said to be equally likely if all of them have the same probability of occurring. Below are some examples of Equally Likely Events.

• Getting 2 or 3 on throwing a die.
• Getting 1, 3, 4 on throwing a die

are all Equally Likely Events since the Probabilities of Each Event are Equal.

Complementary Events

In the Case of Such Events, there will only be two outcomes that state whether an event will occur or not. The complement of an event occurring is the exact opposite that the probability of an event is not occurring.

• It may or may not rain today
• Winning a lottery or not.
• You win the lottery or you don’t.

Probability Density Function

It is a probability function that represents the density of continuous random variables lying between a certain range of values. Standard Normal Distribution is used to create a database or statistics that are used in science to represent the real-valued variables whose values are unknown.

Additive Law of Probability

If E₁ and E₂ be any two events (not necessarily mutually exclusive events), then P(E₁ ∪ E₂) = P(E₁) + P(E₂) – P(E₁ ∩ E₂)

Probability Terms

Some of the Important Probability Terms are discussed here

Sample Space: Set of all possible outcomes that occur in any trail.

Example:

Tossing a Coin, Sample Space (S) = {H, T}

When you Roll a Die Sample Space (S) = {1, 2, 3, 4, 5, 6}

Sample Point: It is one of the Possible Outcome.

Example:

In a deck of cards, 3 of hearts is a sample point.

Experiment or Trial: Series of Actions where outcomes are always uncertain.

Example: Tossing a Coin, Choosing a Card from a Deck of Cards, Throwing a Dice.

Event: Single outcome of an experiment.

Example: Getting Tails while Tossing a Coin is an Event.

Outcome: Possible Result of an Experiment.

Head is a possible outcome when a coin is tossed.

Impossible Event: The Event can’t happen

While Tossing a Coin it is impossible to get head and tail at the same time.

Solved Examples on Probability

1. Find the Probability of getting 2 on rolling a die?

Solution:

Sample Space = {1, 2, 3, 4, 5, 6}

Number of Favourable Events = 1 i.e. {2}

Total Number of Outcomes = 6

Probability P = 1/6

Therefore, Probability of getting 2 on rolling a die is 1/6.

2. Two dice are rolled find the Probability that the Sum is

equal to 1

equal to 5

equal to 8

Solution:

In order to find the probability whose sum is equal to 5 we need to figure out the Sample Space

The post Probability – Definition, Formula, Types, Solved Example Problems appeared first on Learn CBSE.

Time Speed and Distance is a major concept in Mathematics. Time and Distance are used extensively for questions relating to topics like circular motion, boats, and streams, motion in a straight line, clocks, races, etc. This article gives you a complete idea of the Relationship Between Time Speed and Distance, Units, Conversions, etc. Get Formula for Time and Distance, Solved Examples explaining the concept in detail.

Time Speed and Distance – Definition

Speed is a concept in motion that is all about how slow or fast an object travels. Speed is defined as the distance divided by Time. Speed, distance, and time are given to solve for one of the three variables when a piece of certain information is known.

Relationship between Time Speed and Distance

Speed = Distance/Time

Speed tells us how fast or slow an object travels and describes the distance traveled divided by the time taken to cover the distance.

From the above formula, Speed is directly proportional to Distance and inversely proportional to Time.

Units of Time Speed and Distance

Speed Distance and Time has different units for each of them and they are given as under

Time: seconds(s), minutes (min), hours (hr)

Distance: (meters (m), kilometers (km), miles, feet

Speed: m/s, km/hr

If Distance and Time Units are known Speed Units can be derived easily.

Time Speed and Distance Conversions

• To change between km/hour to m/sec, we multiply by 5/18. So, 1 km/hour = 5/18 m/sec
• To change between m/sec to km / hour, we multiply by 18/5. So, 1m /sec = 18/5 km/hour = 3.6 km/hour
• Similarly, 1 km/hr = 5/8 miles/hour
• 1 yard = 3 feet
• 1 mile= 1.609 kilometer
• 1 kilometer= 1000 meters = 0.6214 mile
• 1 mile = 1760 yards
• 1 mile = 5280 feet
• 1 hour= 60 minutes= 60*60 seconds= 3600 seconds
• 1 yard = 3 feet
• 1 mph = (1 x 5280) / (1 x 3600) = 22/15 ft/sec
• 1 mph = (1 x 1760) / (1 x 3600) = 22/45 yards/sec
• For a certain distance, if the ratio of speeds is a : b, then the ratio of times taken to cover the distance is given by b : a and vice versa.

Applications of Time Speed and Distance

Check out different models of questions asked on the concept of Time Speed and Distance. They are as under

Average Speed

Average Speed = Total Distance Travelled/Total Time Taken

Case 1: When Distance is Constant, Average Speed is given by = 2xy/x+y where x, y are two speeds at which the same distance is covered.

Case 2: When Time taken is Constant, Average Speed = (x+y)/2 where x, y are two speeds at which we traveled for the same time.

Examples

1. A person travels from one place to another at 40 km/hr and returns at 160 km/hr. If the total time taken is 5 hours, then find the Distance?

Solution:

Here the Distance is constant, so the Time taken will be inversely proportional to the Speed.

Ratio of Speed = 40:160

= 1:4

Ratio of Time = 4:1

Time taken = 5 hours

Therefore, time taken while going is 4 hours and while returning is 1 hour

Distance = Speed* Time

= 40*4

= 160 Km

Therefore, Distance Travelled is 160 Km.

2. Traveling at 3/2 nd of the original Speed a train is 20 minutes late. Find the usual Time taken by the train to complete the journey?

Solution:

Let the usual Speed be S1 and the usual Time is T₁. As the Distance to be covered in both cases is the same,

The ratio of usual Time to the Time taken when he is late will be the inverse of the usual Speed and the Speed when he is late

If the Speed is S₂ = S₁ then the Time taken T₂ = 3/2 T₁ Given T₂ – T₁ = 20 =>3/2 T1 – T1 = 20 => T1 = 40 minutes.

Inverse Proportionality of Speed & Time

Speed is inversely proportional to time when the distance is constant. If Speeds are in the ratio of m:n then the time taken will be n:m They are two methods of solving questions.

Using Inverse Proportionality
Using Constant Product Rule

Example

After traveling 60km, a train is meeting with an accident and travels at (2/3)rd of the usual Speed and reaches 30 min late. Had the accident happened 15km further on it would have reached 20 min late. Find the usual Speed?

Solution:

Using Inverse Proportionality

Here there are 2 cases

Case 1: accident happens at 60 km

Case 2: accident happens at 75 km

The difference between the two cases is only for the 15 km between 60 and 75. The time difference of 10 minutes is only due to these 15 km.

In case 1, 15 km between 60 and 75 is covered at (2/3)rd Speed.

In case 2, 15 km between 60 and 75 is covered at the usual Speed.

So the usual Time “t” taken to cover 15 km, can be found out as below. 3/2 t – t = 10 mins = > t = 20 mins, d = 15 km

so Usual Speed = 15km/20min = 15km/0.33hr= 45Km/hr

Using Constant Product Rule Method

Let the actual Time taken be T

There is a (1/3)rd decrease in Speed, this will result in a (1/2)nd increase in Time taken as Speed and Time are inversely proportional

The delay due to this decrease is 10 minutes

Thus 1/2 T= 10 and T=20 minutes

Also, Distance = 15 km

Thus Speed = 15/20 minutes = 15km/0.33hr = 45km/hr

Meeting Point

If two people travel from points A and B towards each other they meet at point P. Distance covered by them on the meeting is AB. Time taken by both to meet is the same. Since Time is constant Distance AP and BP will be in the ratio of their Speeds.

Consider the distance between A and B is d.

If two people walking towards each other from A and B. when they meet for the first time they cover a distance of “d ” and when they meet for the second time they cover a distance of “3d” and when they meet for the third time they cover a distance of “5d” …..

Example

1. Amar and Arun have to travel from Delhi to Jaipur in their respective cars. Arun is driving at 45 kmph while Amar is driving at 60 kmph. Find the Time taken by Amar to reach Jaipur if Arun takes 6 hrs?

Solution:

Since the Distance covered is constant in both cases, the Time taken will be inversely proportional to the Speed.

From the given data, the Speed of Amar and Arun are in ratio 45:60 or 3:4.

So the ratio of the Time taken by Arun to that taken by Amar will be in the ratio 4:3. So if Arun takes 6 hrs, Amar will take 4.5 hrs.

Solved Examples on Time and Distance

1. Seetha is driving a car with a speed of 60 km/hr for 1.5hr. How much distance does she travel?

Solution:

Speed = 60 Km/hr

Time = 1.5 hr

Distance = Speed *Time

= 60*1.5

= 90 Km

Therefore, Sheela Travels a distance of 90km.

2. While going to an office, Ram travels at a speed of 35 kmph, and on his way back, he travels at a speed of 40 kmph. What is his average speed for the whole journey?

Solution:

In this case, Distance is constant

Average Speed = 2xy/x+y where x, y is the speeds at which the distance is covered

Substitute the Speeds from the given data

Average Speed = (2*35*40)/35+40

= 37.33 km/hr

The Average Speed of the Whole Journey is 37.33Kmph

3. Ramu and Somesh are standing at two ends of a room with a width of 40 m. They start walking towards each other along the width of the room with a Speed of 4 m/s and 3 m/s respectively. Find the total distance traveled by Ramu when he meets Somesh for the third time?

Solution:

When Ramu meets Somesh for the third time he would have covered a distance of 5d i.e. 5*40m = 200m

The ratio of Speed of Ramu and Somesh is 4:3 so the Distance traveled by both of them will also be in the ratio of 4:3

The post Time and Distance – Formula, Units, Conversions, Relationship between Time Speed and Distance appeared first on Learn CBSE.

Learn What is meant by Speed and How to Calculate the Motion or Speed by going through the entire article. Check out Formula to find the Speed of a Train when Train Passes through a Stationary Object and Train Passing through a Stationary Object of Certain Length. We have mentioned Solved Examples explaining step by step for a better understanding of the concept. Learn about Time and Distance Concepts too from here and get a good hold of the concept by practicing the sample problems available on a regular basis.

How to find the Speed of a Train?

There are two different scenarios to calculate the Speed of a Train. We explained each scenario in detail by taking a few examples. Understand the concept behind them easily. They are as under

• Train Passes through a Stationary Object
• Train Passes through a Stationary Object having Some Length

When a Train Passes through a Stationary Object

Consider the length of the train along with the engine be x. When the end of the train passes the object then the engine of the train travels the distance equal to the train length.

Time taken by train to pass the stationary object = length of the train/speed of the train

When a Train Passes through a Stationary Object having Some Length

When the end of the train passes the stationary object having a certain length, then the engine of the train moves a distance equal to the sum of both the length of the train and the stationary object.

Time taken by the train to pass stationary object = (length of the train+length of the stationary object)/speed of the train

Solved Examples on Speed of a Train

1. Find the time taken by a train 125 m long, running at a speed of 108 km/hr in crossing the pole?

Solution:

Length of the Train = 125m

Speed of Train = 108 km/hr

= 108*5/18

= 30 m/sec

Time taken by train to cross the pole = 125m/30m/sec

= 4.1 sec

2. A train 320 m long is running at a speed of 60 km/hr. what time will it take to cross a 160 m long tunnel?

Solution:

Length of the train = 320m

Length of the tunnel = 160m

Length of Train+Length of Tunnel = (320+160) = 480m

Speed of train = 60 km/hr

= 60*5/18

= 16.66 m/sec

Time taken by train to cross the tunnel = 480m/16.66m/sec = 28.8 sec

3. A train is running at a speed of 75 km/hr. if it crosses a pole in just 15 second, what is the length of the train?

Solution:

Speed of the train = 75 km/hr

Speed of the train = 75 × 5/18 m/sec = 20.83 m/sec

Time taken by the train to cross the pole = 15 seconds

Therefore, length of the train = 20.83 m/sec × 15 sec = 312.5 m

4. A train 250 m long crosses the bridge 120 m in 20 seconds. Find the speed of the train in km/hr?

Solution:

Length of the train = 250m

Length of the Bridge = 120 m

Total Length = Length of Train + Lenth of Bridge

= 250+120

= 370 m

Time taken to cross the bridge = 20 seconds

Speed of the Train = Length/Time = 370m/20 sec = 18.5 m/sec

To convert m/sec to km/hr multiply with 18/5

Speed of the Train in km/hr = 18.5*18/5

= 66.6 km/hr

Therefore, the speed of the train is 66.6 km/hr

The post Speed of Train | How to Calculate the Motion or Speed of a Train? appeared first on Learn CBSE.

In this article, we will learn the mathematical relation existing between Speed Distance and Time. Speed is the distance traveled by a moving object in unit time. Go through the entire article to learn about How is Speed Related to Time and Distance. Check out Solved Problems for finding Speed Time and Distance if few parameters are known. To help you understand the concept better we have provided Detailed Solutions.

If the distance is in km and the time taken to cover it is in hrs then the unit of Speed is given by km/hr. In the same way, if the distance is in m and the time taken to cover it is in sec then the unit of speed is given by m/sec. Speed can be either uniform or variable.

Example: If a Car travels 60 km in 1 hr then the Speed of it traveled is given by 60km/hr.

Types of Speed

There are two different types of Speeds and each one of their definition along with examples is explained in detail.

• Uniform Speed
• Variable Speed
• Average Speed

Uniform Speed: If an object travels the same distance in the same intervals of time then the object is said to be traveling with a Uniform Speed.

Example: If a car covers 60 km in 1st hour and 60 km in the 2nd hour, 60 km in the 3rd hour then the car is said to be moving with a uniform speed of 60kmph or 60 km/hr.

Variable Speed: If an object travels a different distance in the same intervals of time then the object is said to be moving with variable speed.

Example: If a car travels at 45 km for the 1st hour and 54 km for the 2nd hour and 65 km in the third hour then the car has a variable speed.

Average Speed: If a moving object travels different distances d₁, d₂, d₃, …. d_n with different speeds V₁, V₂, ……, V_n m/sec in time t₁, t₂, …., t_n seconds.

Average Speed of the Body = Total Distance Traveled/Total Time Taken

= d₁+d₂+d₃…..d_n/t₁+t₂+….t_n

Relation between Time Speed and Distance is given by the following

Speed = Distance/Time

Distance = Speed *Time

Time = Distance/Speed

Solved Problems on Relationship between Time Speed and Distance

1. A car travels a distance of 400 km in 5 hours. What is its speed in km/hr?

Solution:

We know the formula for Speed = Distance/Time

Speed = 400 km/5 hr

= 80 km/hr

The speed at which a car travels is 80 km/hr.

2. The distance between the two stations is 240 km. A train takes 2 hours to cover this distance. Calculate the speed of the train in km/hr and m/s?

Solution:

Distance between two stations = 240 km

Time = 2 hrs

Speed = Distance/Time

= 240 km/2 hr

= 120 km/hr

Speed of the train in m/sec is obtained by multiplying with 5/18

= 120*5/18

= 33.33 m/sec

3. Traveling at a speed of 45 kmph, how long is it going to take to travel 90 km?

Solution:

Speed = 45 kmph

Distance = 90 Km

we know the relation between speed distance and time is Speed = Distance/Time

45 kmph = 90 km/Time

Time = 90 km/45 kmph

= 2 hours

The post Relationship between Speed, Distance and Time | How is Speed Related to Time and Distance? appeared first on Learn CBSE.

In Maths Mensuration is nothing but a measurement of 2-D and 3-D Geometrical Figures. Mensuration is the study of the measurement of shapes and figures. We can measure the area, perimeter, and volume of geometrical shapes such as Cube, Cylinder, Cone, Cuboid, Sphere, and so on.

Keep reading this page to learn deeply about the mensuration. We can solve the problems easily, if and only we know the formulas of the particular shape or figure. This article helps to learn the mensuration formulae with examples. Learn the difference between the 2-D and 3-D shapes from here. Understand the concept of Mensuration by using various formulas.

Definition of Mensuration

Mensuration is the theory of measurement. It is the branch of mathematics that is used for the measurement of various figures like the cube, cuboid, square, rectangle, cylinder, etc. We can measure the 2 Dimensional and 3 Dimensional figures in the form of Area, Perimeter, Surface Area, Volume, etc.

What is a 2-D Shape?

The shape or figure with two dimensions like length and width is known as the 2-D shape. An example of a 2-D figure is a Square, Rectangle, Triangle, Parallelogram, Trapezium, Rhombus, etc. We can measure the 2-D shapes in the form of Area (A) and Perimeter (P).

What is 3-D Shape?

The shape with more or than two dimensions such as length, width, and height then it is known as 3-D figures. Examples of 3-Dimensional figures are Cube, Cuboid, Sphere, Cylinder, Cone, etc. The 3D figure is determined in the form of Total Surface Area (TSA), Lateral Surface Area (LSA), Curved Surface Area (CSA), and Volume (V).

Introduction to Mensuration

The important terminologies that are used in mensuration are Area, Perimeter, Volume, TSA, CSA, LSA.

• Area: The Area is an extent of two-dimensional figures that measure the space occupied by the closed figure. The units for Area is square units. The abbreviation for Area is A.
• Perimeter: The perimeter is used to measure the boundary of the closed planar figure. The units for Perimeter is cm or m. The abbreviation for Perimeter is P.
• Total Surface Area: The total surface area is the combination or sum of both lateral surface area and curved surface area. The units for the total surface area is square cm or m. The abbreviation for the total surface area is TSA.
• Lateral Surface Area: It is the measure of all sides of the object excluding top and base. The units for the lateral surface area is square cm or m. The abbreviation for the lateral surface area is LSA.
• Curved Surface Area: The area of a curved surface is called a Curved Surface Area. The units of the curved surface area are square cm or m. The abbreviation for the curved surface area is CSA.
• Volume: Volume is the measure of the three dimensional closed surfaces. The units for volume is cubic cm or m. The abbreviation for Volume is V.

Mensuration Formulas for 2-D Figures

Check out the formulas of 2-dimensional figures from here. By using these mensuration formulae students can easily solve the problems of 2D figures.

1. Rectangle:

• Area = length × width
• Perimeter = 2(l + w)

2. Square:

• Area = side × side
• Perimeter = 4 × side

3. Circle:

• Area = Πr²
• Circumference = 2Πr
• Diameter = 2r

4. Triangle:

• Area = 1/2 × base × height
• Perimeter = a + b + c

5. Isosceles Triangle:

• Area = 1/2 × base × height
• Perimeter = 2 × (a + b)

6. Scalene Triangle:

• Area = 1/2 × base × height
• Perimeter = a + b + c

7. Right Angled Triangle:

• Area = 1/2 × base × height
• Perimeter = b + h + hypotenuse
• Hypotenuse c = a²+b²

8. Parallelogram:

• Area = a × b
• Perimeter = 2(l + b)

9. Rhombus:

• Area = 1/2 × d1 × d2
• Perimeter = 4 × side

10. Trapezium:

• Area = 1/2 × h(a + b)
• Perimeter = a + b + c + d

11. Equilateral Triangle:

• Area = √3/4 × a²
• Perimeter = 3a

Mensuration Formulas of 3D Figures

The list of the mensuration formulae for 3-dimensional shapes is given below. Learn the relationship between the various parameters from here.

1. Cube:

• Lateral Surface Area = 4a²
• Total Surface Area = 6a²
• Volume = a³

2. Cuboid:

• Lateral Surface Area = 2h(l + b)
• Total Surface Area = 2(lb + bh + lh)
• Volume = length × breadth × height

3. Cylinder:

• Lateral Surface Area = 2Πrh
• Total Surface Area = 2Πrh + 2Πr²
• Volume = Πr²h

4. Cone:

• Lateral Surface Area = Πrl
• Total Surface Area = Πr(r + l)
• Volume = 1/3 Πr²h

5. Sphere:

• Lateral Surface Area = 4Πr²
• Total Surface Area = 4Πr²
• Volume = (4/3)Πr³

6. Hemisphere:

• Lateral Surface Area = 2Πr²
• Total Surface Area = 3Πr²
• Volume = (2/3)Πr³

Solved Problems on Mensuration

Here are some questions that help you to understand the concept of Mensuration. Use the Mensuration formulas to solve the problems.

1. Find the Length of the Rectangle whose Perimeter is 24 cm and Width is 3 cm?

Solution:

Given that,
Perimeter = 24 cm
Width = 3 cm
Perimeter of the rectangle = 2(l + w)
24 cm = 2(l + 3 cm)
2l + 6 = 24
2l + 6 = 24
2l = 24 – 6 = 18
2l = 18
l = 9 cm
Thus length of the rectangle = 9 cm

2. Calculate the volume of the Cuboid whole base area is 60 cm² and height is 5 cm.

Solution:

Given,
Base area = 60 cm²
Height = 5 cm
Volume of the Cuboid = base area × height
V = 60 cm² × 5 cm
V = 300 cm³
Thus the volume of the cuboid is 300 cm³.

3. Find the area of the Cube whose side is 10 centimeters.

Solution:

Given, side = 10 cm
Lateral Surface Area = 4a²
LSA = 4 × 10 × 10 = 400 cm²
Total Surface Area = 6a²
= 6 × 10 × 10 = 600 cm²
Volume of the cube = a³
V = 10 × 10 × 10 = 1000 cm³
Therefore the volume of the cube is 1000 cubic centimeters.

4. What is the lateral surface area of the sphere if the radius is 5 cm.

Solution:

Given,
The radius of the sphere = 5 cm
The formula for LSA of sphere = 4Πr²
Π = 3.14 or 22/7
LSA = 4 × 3.14 × 5 cm × 5 cm
LSA = 314 sq. cm
Thus the lateral surface area of the sphere is 314 sq. cm

5. What is the area of the parallelogram if the base is 15 cm and height is 10 cm.

Solution:

Given, Base = 15 cm
Height = 10 cm
We know that,
Area of parallelogram = bh
A = 15 cm × 10 cm
A = 150 sq. cm
Therefore the area of the parallelogram is 150 sq. cm.

FAQs on Mensuration

1. What is the use of Mensuration?

Mensuration is used to find the length, area, perimeter, and volume of the geometric figures.

2. What is the difference between 2D and 3D figures?

In 2D we can measure the area and perimeter. In 3D we can measure LSA, TSA, and Volume.

3. What is Mensuration in Math?

Mensuration is the branch of mathematics that studies the theory of measurement of 2D and 3D geometric figures or shapes.

The post Mensuration – Definition, Introduction, Formulas, Solved Problems appeared first on Learn CBSE.

Area and Perimeter is an important and basic topic in the Mensuration of 2-D or Planar Figures. The area is used to measure the space occupied by the planar figures. The perimeter is used to measure the boundaries of the closed figures. In Mathematics, these are two major formulas to solve the problems in the 2-dimensional shapes.

Each and every shape has two properties that are Area and Perimeter. Students can find the area and perimeter of different shapes like Circle, Rectangle, Square, Parallelogram, Rhombus, Trapezium, Quadrilateral, Pentagon, Hexagon, and Octagon. The properties of the figures will vary based on their structures, angles, and size. Scroll down this page to learn deeply about the area and perimeter of all the two-dimensional shapes.

Area and Perimeter Definition

Area: Area is defined as the measure of the space enclosed by the planar figure or shape. The Units to measure the area of the closed figure is square centimeters or meters.

Perimeter: Perimeter is defined as the measure of the length of the boundary of the two-dimensional planar figure. The units to measure the perimeter of the closed figures is centimeters or meters.

Formulas for Area and Perimeter of 2-D Shapes

1. Area and Perimeter of Rectangle:

• Area = l × b
• Perimeter = 2 (l + b)
• Diagnol = √l² + b²

Where, l = length
b = breadth

2. Area and Perimeter of Square:

• Area = s × s
• Perimeter = 4s

Where s = side of the square

3. Area and Perimeter of Parallelogram:

• Area = bh
• Perimeter = 2( b + h)

Where, b = base
h = height

4. Area and Perimeter of Trapezoid:

• Area = 1/2 × h (a + b)
• Perimeter = a + b + c + d

Where, a, b, c, d are the sides of the trapezoid
h is the height of the trapezoid

5. Area and Perimeter of Triangle:

• Area = 1/2 × b × h
• Perimeter = a + b + c

Where, b = base
h = height
a, b, c are the sides of the triangle

6. Area and Perimeter of Pentagon:

• Area = (5/2) s × a
• Perimeter = 5s

Where s is the side of the pentagon
a is the length

7. Area and Perimeter of Hexagon:

• Area = 1/2 × P × a
• Perimeter = s + s + s + s + s + s = 6s

Where s is the side of the hexagon.

8. Area and Perimeter of Rhombus:

• Area = 1/2 (d1 + d2)
• Perimeter = 4a

Where d1 and d2 are the diagonals of the rhombus
a is the side of the rhombus

9. Area and Perimeter of Circle:

• Area = Πr²
• Circumference of the circle = 2Πr

Where r is the radius of the circle
Π = 3.14 or 22/7

10. Area and Perimeter of Octagon:

• Area = 2(1 + √2) s²
• Perimeter = 8s

Where s is the side of the octagon.

Solved Examples on Area and Perimeter

Here are some of the examples of the area and perimeter of the geometric figures. Students can easily understand the concept of the area and perimeter with the help of these problems.

1. Find the area and perimeter of the rectangle whose length is 8m and breadth is 4m?

Solution:

Given,
l = 8m
b = 4m
Area of the rectangle = l × b
A = 8m × 4m
A = 32 sq. meters
The perimeter of the rectangle = 2(l + b)
P = 2(8m + 4m)
P = 2(12m)
P = 24 meters
Therefore the area and perimeter of the rectangle is 32 sq. m and 24 meters.

2. Calculate the area of the rhombus whose diagonals are 6 cm and 5 cm?

Solution:

Given,
d1 = 6cm
d2 = 5 cm
Area = 1/2 (d1 + d2)
A = 1/2 (6 cm + 5cm)
A = 1/2 × 11 cm
A = 5.5 sq. cm
Thus the area of the rhombus is 5.5 sq. cm

3. Find the area of the triangle whose base and height are 11 cm and 7 cm?

Solution:

Given,
Base = 11 cm
Height = 7 cm
We know that
Area of the triangle = 1/2 × b × h
A = 1/2 × 11 cm × 7 cm
A = 1/2 × 77 sq. cm
A = 38.5 sq. cm
Thus the area of the triangle is 38.5 sq. cm.

4. Find the area of the circle whose radius is 7 cm?

Solution:

Given,
Radius = 7 cm
We know that,
Area of the circle = Πr²
Π = 3.14
A = 3.14 × 7 cm × 7 cm
A = 3.14 × 49 sq. cm
A = 153.86 sq. cm
Therefore the area of the circle is 153.86 sq. cm.

5. Find the area of the trapezoid if the length, breadth, and height is 8 cm, 4 cm, and 5 cm?

Solution:

Given,
a = 8 cm
b = 4 cm
h = 5 cm
We know that,
Area of the trapezoid = 1/2 × h(a + b)
A = 1/2 × (8 + 4)5
A = 1/2 × 12 × 5
A = 6 cm× 5 cm
A = 30 sq. cm
Therefore the area of the trapezoid is 30 sq. cm.

6. Find the perimeter of the pentagon whose side is 5 meters?

Solution:

Given that,
Side = 5 m
The perimeter of the pentagon = 5s
P = 5 × 5 m
P = 25 meters
Therefore the perimeter of the pentagon is 25 meters.

FAQs on Area and Perimeter

1. How does Perimeter relate to Area?

The perimeter is the boundary of the closed figure whereas the area is the space occupied by the planar.

2. How to calculate the perimeter?

The perimeter can be calculated by adding the lengths of all the sides of the figure.

3. What is the formula for perimeter?

The formula for perimeter is the sum of all the sides.

The post Area and Perimeter Definition, Formulas | How to find Area and Perimeter? appeared first on Learn CBSE.

NCERT Solutions for Class 8 Maths Chapter 2 Linear Equations in One Variable Ex 2.1

• Class 8 Maths Linear Equations in One Variable Exercise 2.1
• Class 8 Maths Linear Equations in One Variable Exercise 2.2
• Class 8 Maths Linear Equations in One Variable Exercise 2.3
• Class 8 Maths Linear Equations in One Variable Exercise 2.4
• Class 8 Maths Linear Equations in One Variable Exercise 2.5
• Class 8 Maths Linear Equations in One Variable Exercise 2.6
• Linear Equations in One Variable Class 8 Extra Questions

NCERT Solutions for Class 8 Maths Chapter 2 Linear Equations in One Variable Exercise 2.1

Ex 2.1 Class 8 Maths Question 1.
Solve the equation: x – 2 = 7.
Solution:
Given: x – 2 = 7
⇒ x – 2 + 2 = 7 + 2 (adding 2 on both sides)
⇒ x = 9 (Required solution)

Ex 2.1 Class 8 Maths Question 2.
Solve the equation: y + 3 = 10.
Given: y + 3 = 10
⇒ y + 3 – 3 = 10 – 3 (subtracting 3 from each side)
⇒ y = 7 (Required solution)

Ex 2.1 Class 8 Maths Question 3.
Solve the equation: 6 = z + 2
Solution:
We have 6 = z + 2
⇒ 6 – 2 = z + 2 – 2 (subtracting 2 from each side)
⇒ 4 = z
Thus, z = 4 is the required solution.

Ex 2.1 Class 8 Maths Question 4.
Solve the equations: \(\frac { 3 }{ 7 }\) + x = \(\frac { 17 }{ 7 }\)
Solution:

Ex 2.1 Class 8 Maths Question 5.
Solve the equation 6x = 12.
Solution:
We have 6x = 12
⇒ 6x ÷ 6 = 12 ÷ 6 (dividing each side by 6)
⇒ x = 2
Thus, x = 2 is the required solution.

Ex 2.1 Class 8 Maths Question 6.
Solve the equation \(\frac { t }{ 5 }\) = 10.
Solution:
Given \(\frac { t }{ 5 }\) = 10
⇒ \(\frac { t }{ 5 }\) × 5 = 10 × 5 (multiplying both sides by 5)
⇒ t = 50
Thus, t = 50 is the required solution.

Ex 2.1 Class 8 Maths Question 7.
Solve the equation \(\frac { 2x }{ 3 }\) = 18.
Solution:
We have \(\frac { 2x }{ 3 }\) = 18
⇒ \(\frac { 2x }{ 3 }\) × 3 = 18 × 3 (multiplying both sides by 3)
⇒ 2x = 54
⇒ 2x ÷ 2 = 54 ÷ 2 (dividing both sides by 2)
⇒ x = 27
Thus, x = 27 is the required solution.

Ex 2.1 Class 8 Maths Question 8.
Solve the equation 1.6 = \(\frac { y }{ 1.5 }\)
Solution:
Given: 1.6 = \(\frac { y }{ 1.5 }\)
⇒ 1.6 × 1.5 = \(\frac { y }{ 1.5 }\) × 1.5 (multiplying both sides by 1.5)
⇒ 2.40 = y
Thus, y = 2.40 is the required solution.

Ex 2.1 Class 8 Maths Question 9.
Solve the equation 7x – 9 = 16.
Solution:
We have 7x – 9 = 16
⇒ 7x – 9 + 9 = 16 + 9 (adding 9 to both sides)
⇒ 7x = 25
⇒ 7x ÷ 7 = 25 ÷ 7 (dividing both sides by 7)
⇒ x = \(\frac { 25 }{ 7 }\)
Thus, x = \(\frac { 25 }{ 7 }\) is the required solution.

Ex 2.1 Class 8 Maths Question 10.
Solve the equation 14y – 8 = 13.
Solution:
We have 14y – 8 = 13
⇒ 14y – 8 + 8 = 13 + 8 (adding 8 to both sides)
⇒ 14y = 21
⇒ 14y ÷ 14 = 21 ÷ 14 (dividing both sides by 14)
⇒ y = \(\frac { 21 }{ 14 }\)
⇒ y = \(\frac { 3 }{ 2 }\)
Thus, y = \(\frac { 3 }{ 2 }\) is the required solution.

Ex 2.1 Class 8 Maths Question 11.
Solve the equation 17 + 6p = 9.
Solution:
We have, 17 + 6p = 9
⇒ 17 – 17 + 6p = 9 – 17 (subtracting 17 from both sides)
⇒ 6p = -8
⇒ 6p ÷ 6 = -8 ÷ 6 (dividing both sides by 6)
⇒ p = \(\frac { -8 }{ 6 }\)
⇒ p = \(\frac { -4 }{ 3 }\)
Thus, p = \(\frac { -4 }{ 3 }\) is the required solution.

Ex 2.1 Class 8 Maths Question 12.
Solve the equation \(\frac { x }{ 3 }\) + 1 = \(\frac { 7 }{ 15 }\)
Solution:

More CBSE Class 8 Study Material

• NCERT Solutions for Class 8 Maths
• NCERT Solutions for Class 8 Science
• NCERT Solutions for Class 8 Social Science
• NCERT Solutions for Class 8 English
• NCERT Solutions for Class 8 English Honeydew
• NCERT Solutions for Class 8 English It So Happened
• NCERT Solutions for Class 8 Hindi
• NCERT Solutions for Class 8 Sanskrit
• NCERT Solutions

The post NCERT Solutions for Class 8 Maths Chapter 2 Linear Equations in One Variable appeared first on Learn CBSE.

NCERT Solutions for Class 12 Sanskrit Bhaswati: Detailed, Step-by-Step NCERT Solutions for Class 12 Sanskrit Bhaswati भास्वती भाग 2 Text Book Questions and Answers.

Class 12 Sanskrit NCERT Solutions | Bhaswati Class 12 Guide

Don’t you have a hardcopy of the Sanskrit Textbook and worried about how to learn it. Don’t worry as we have curated the NCERT Solutions for Class 12 Sanskrit in a simple and easy to understand language. You can rely on the Class 12 NCERT Solutions for Sanskrit provided here as they are given to you after ample research. Make the most out of the NCERT Solutions prevailing as per the latest CBSE Guidelines and score high in your board exams. Download the Sanskrit 12th Class NCERT Solutions PDF free of cost through the quick links available.

Bhaswati Sanskrit Book Class 12 Solutions

Take the help of the Chapterwise NCERT Solutions of Class 12 Sanskrit and ace up your preparation. Enhance Subject Knowledge on Sanskrit through the detailed solutions provided. All the Solutions are provided in a clear manner so that students can understand them quickly and clarify their doubts. Simply click on the quick links available below and prepare the concepts underlying accordingly.

खण्डः क

• अपठितांशावबोधनम्

खण्डः ख – संस्कृतेन रचनात्मकं लिखितकार्यम्

• पत्र-लेखनम् (अनौपचारिकं पत्रम्/प्रार्थनापत्रम्)
• लघुकथा-लेखनम् (शब्दसूचीसाहाय्येन, रिक्तस्थानपूर्ति-माध्यमेन)
• सङ्केताधारितम् अनुच्छेदलेखनम्

खण्डः ग
अनुप्रयुक्तव्याकरणम्

• सन्धि-प्रकरण
• समास-प्रकरण
• प्रकृति-प्रत्यय-विभाग
• अन्विति-प्रकरण
• उपपदविभक्ति प्रयोग

खण्डः घ
भाग I – पठितांश-अवबोधनम्

• Chapter 1 उत्तिष्ठत जाग्रत (उठो, जागो)
• Chapter 2 सूर्यः एव प्रकृतेः आधारः (सूर्य ही पृथ्वी का आधार है)
• Chapter 3 राष्ट्रचिन्ता गरीयसी (राष्ट्र की चिन्ता अधिक महत्त्वपूर्ण है)
• Chapter 4 दूरदृष्टिः फलप्रदा (दूरदृष्टि अच्छे परिणाम देती है)
• Chapter 5 अहो! राजते कीदृशीयं हिमानी (अहा! कैसी सुशोभित है यह हिम-घाटी)
• Chapter 6 सुधामुचः वाचः (अमृत बरसाने वाले वचन)
• Chapter 7 दारिद्र्ये दुर्लभं सत्त्वम् (दरिद्रता में मनोबल का होना दुर्लभ है)
• Chapter 8 आश्चर्यमयं विज्ञानजगत् (आश्चर्यों से भरा है विज्ञान का यह संसार)

भाग II – सामान्यः संस्कृतसाहित्यपरिचयः

• पाठ्यपुस्तके संकलितपाठ्यांशानाम् कवीनां कृतीनां संस्कृतेन परिचयः
  • लेखकानां देश-काल-ग्रन्थाणाम्
  • कवि/लेखक परिचयः
• संस्कृते गद्य-पद्य-नाटकादिविधानां मुख्यविशेषतानां परिचयः

CBSE Class 12 Sanskrit Syllabus

संस्कृतम् कक्षा – 12
पूर्णाङ्काः 100
एकम् प्रश्नपत्रम्

अस्मिन् प्रश्नपत्रे चत्वारःः खण्डाः भविष्यन्ति
खण्डः “क” अपठितांश-अवबोधनम् (10)
खण्डः “ख” रचनात्मककार्यम् (15)
खण्डः “ग” अनुप्रयुक्तव्याकरणम् (30)
खण्डः “घ” (45)
(अ) पठित-अवबोधनम् (35)
(ब) संस्कृतसाहित्येतिहास्य परिचयः (10)

प्रतिखण्डं विस्तृतविवरणम्
खण्डः ‘क’ – (अपठितांशअवबोधनम्)

80-100 शब्दपरिमितः एक सरलः अपठितः गद्यांशः। प्रश्नवैविध्यम् (अङ्काः – 10, कालांशः – 21)
(i) एकपदेन उत्तरम्
(ii) पूर्णवाक्येन उत्तरम्
(iii) सर्वनामस्थाने संज्ञाप्रयोगः
(iv) कर्तृक्रिया-पदचयनम्
(v) विशेषण-विशेष्य/पर्याय/विलोमादिचयनम्
(vi) समुचितशीर्षकप्रदानम्

खण्डः ‘ख’
(संस्कृतेन रचनात्मकं लिखितकार्यम्) (अङ्काः – 15, कालांशः – 32)

1. अनौपचारिकं पत्रम्/प्रार्थनापत्रम्
2. लघुकथा (शब्दसूचीसाहाय्येन, रिक्तस्थानपूर्ति-माध्यमेन)
3. संकेताधारितम् अनुच्छेदलेखनम् (चित्रमधिकृत्य, निर्दिष्टशब्दसूची-साहाय्येन)

खण्डः ‘ग’
(अनुप्रयुक्तव्याकरणम्) (अङ्काः – 30, कालांशः – 63)

(i) पाठाधारिताः सन्धिच्छेदाः (2 + 2 + 2 = 6)
स्वरसन्धिः, व्यंजनसन्धिः, विसर्गसन्धिः
(ii) पाठाधारितसमस्तपदानां विग्रहा: (6)
अव्ययीभावः, द्विगुः, द्वन्द्वः, तत्पुरुषः, कर्मधारयः, बहुव्रीहिः
(iii) प्रत्ययाः
अधोलिखितप्रत्यययोगेन वाक्यसंयोजनम्/सङ्केताधारितरिक्तस्थानपूर्तिः
(अ) कृत् – क्त, क्तवतु, क्त्वा, तुमुन्, ल्यप्, तव्यत्, अनीयर्, क्तिन्, शतृ, शान। (5)
(आ) तद्धित — मतुप्, इन्, ठक्, ठञ्, त्व, तल्। (3)
(iv) अन्वितिः (5)
कर्तृ-क्रिया-अन्वितिः/विशेषण-विशेष्य-अन्वितिः
(v) उपपदविभक्तिप्रयोगः (पाठ्यपुस्तकम् आधृत्य) (5)

खण्डः ‘घ’
भागः I – (पठितांश-अवबोधनम् ) (अङ्काः – 45, कालांशः – 94)

(अ) अंशत्रयम् (15)
(i) एकः गद्यांशः (5)
(ii) एकः नाट्यांशः (5)
(iii) एकः पद्यांश: (5)
प्रश्नवैविध्यम्
(i) एकपदेन उत्तरम् (1)
(ii) पूर्णवाक्येन उत्तरम् (1)
(iii) विशेषण-विशेष्य-अन्विति:/पर्याय/विलोमचयनम् (1)
(iv) सर्वनामस्थाने संज्ञाप्रयोगः (1)
(v) कर्तृ-क्रिया-पदचयनम् (1)
(आ) (i) उद्धृतांशानम् प्रसङ्गसन्दर्भलेखनम् कः कम् कथयति/सन्दर्भग्रन्थस्य लेखकस्य च नामोल्लेखनम् (4)
(ii) प्रदत्ते भावार्थेत्रये शुद्धभावार्थचयनम्/ प्रदत्ते भावार्थे रिक्तस्थानपूर्तिः (4)
(iii) उद्धृतश्लोकानाम् अन्वयेषु रिक्तस्थानपूर्तिः (4)
(iv) प्रदत्तवाक्यानां क्रमयोजनम् (4)
(v) प्रदत्तपंक्तिषु प्रसङ्गानुसारं श्लिष्टपदानाम्/पदानाम् अर्थलेखनम् (4)

भागः II – (सामान्यः संस्कृतसाहित्यपरिचयः) (10)
1. (अ) पाठ्यपुस्तके संकलितपाठ्यांशानां कवीनां कृतीनां संस्कृतेन परिचयः (1 × 5 = 5)
(आ) संस्कृते गद्य-पद्य-नाटकादिविधानां मुख्यविशेषतानां परिचयः (5)

Benefits of 12th Class NCERT Solutions on Sanskrit

Go through the advantages of referring to Class 12th NCERT Solutions on Sanskrit from the below sections. They are along the lines

• Students can learn all the concepts in a simple and easy to understand language.
• All the Solutions provided are available for download and you can access them free of cost.
• Lay a stronger foundation of basics by solving the NCERT Solutions provided.
• Detailed Solutions provided are given by subject experts and as per the latest syllabus guidelines.

FAQs on Class 12 Sanskrit NCERT Solutions

1. How to download NCERT Solutions for Class 12 Sanskrit?

You can download NCERT Solutions for Class 12 Sanskrit by simply tapping on the quick links available. Once you click on them you will be directed to a new page having the download option. Tap on that and save it for future reference.

2. From where students can get detailed NCERT Solutions for Class 12 Sanskrit?

Students can get detailed NCERT Solutions for Class 12 Sanskrit on our page.

3. How to study 12th Grade Sanskrit Concepts easily?

Take the help of NCERT Solutions for 12th Grade Sanskrit to prepare the concepts of Sanskrit easily.

Final words

We wish the data shed above regarding NCERT Solutions for Class 12 Sanskrit has been beneficial to an extent. If you have any other queries feel free to reach us and we will get back to you at the earliest possibility. Bookmark our site for the latest updates on NCERT Solutions of various classes at your fingertips.

The post NCERT Solutions for Class 12 Sanskrit Bhaswati भास्वती भाग 2 | कक्षा 12 संस्कृत गाइड appeared first on Learn CBSE.

Solved CBSE Sample Paper 2020-2021 Class 10 Maths Standard and Basic with Solutions: Solving Pre Board CBSE Sample Papers for Class 10 Maths Standard and Basic with Solutions Answers 2020-2021 Pdf Download to understand the pattern of questions ask in the board exam. Know about the important concepts to be prepared for CBSE Class 10 Maths board exam and Score More marks. Here we have given CBSE Class 10 Maths Sample Papers. According to new CBSE Exam Pattern, MCQ Questions for Class 10 Maths Carries 20 Marks.

Board – Central Board of Secondary Education
Subject – CBSE Class 10 Maths
Year of Examination – 2020, 2019, 2018, 2017, 2016.

CBSE Sample Paper 2021 Class 10 Maths Standard and Basic with Solutions

CBSE Sample Paper 2021 Class 10 Maths Standard with Solutions

• CBSE Sample Paper 2020 Class 10 Maths Standard with Solution Set 1
• Maths Sample Paper Class 10 2020 Standard Solution Set 2
• Sample Paper Class 10 Maths Standard with Solution Set 3
• CBSE Class 10 Maths Standard Sample Paper Set 4 for Practice
• Sample Paper of Maths Standard Class 10 Set 5 for Practice

CBSE Sample Paper 2020-2021 Class 10 Maths Basic with Solutions

• CBSE Sample Paper 2020 Class 10 Maths Basic with Solution Set 1
• Class 10 Maths Basic Sample Paper 2020 Solved Set 2
• Sample Paper of Class 10 Maths Basic Set 3
• Maths Basic Sample Paper Class 10 Set 4 for Practice
• Sample Paper for Class 10 Maths Basic Set 5 for Practice

Download Formula Book for Class 10 Maths and Science

CBSE Sample Papers 2020 Maths Basic, Maths Standard, Science, Social Science, Hindi, English, and Sanskrit

How To Take CBSE Class 10 Maths Sample Papers?

Step 1 – Download the CBSE Class 10 Sample Question Papers or Pre Board Model Papers or Previous year question papers that you want to take.

Step 2 – Take the exam seriously just like you would take the real exam.

Step 3 – Evaluate your paper – mark the questions you couldn’t answer or get incorrect.

Step 4 – Revise the related concepts and topics.

Solved CBSE Sample Papers for Class 10 Maths 2019

Solved CBSE Sample Papers for Class 10 for 2018-19 are tabulated as follows:

CBSE Previous Year Question Papers Class 10 Maths With Solutions

CBSE Previous Year Question Papers Class 10 Maths With Solutions are tabulated as follows:

• CBSE Previous Year Question Papers Class 10 Maths 2019 Outside Delhi
• CBSE Previous Year Question Papers Class 10 Maths 2019 Delhi
• CBSE Previous Year Question Papers Class 10 Maths 2018
• CBSE Previous Year Question Papers Class 10 Maths 2017 Outside Delhi Term 2
• CBSE Previous Year Question Papers Class 10 Maths 2017 Delhi Term 2
• CBSE Previous Year Question Papers Class 10 Maths 2016 Delhi Term 2
• CBSE Previous Year Question Papers Class 10 Maths 2015 Delhi Term 2
• CBSE Previous Year Question Papers Class 10 Maths 2014 Delhi Term 2
• CBSE Previous Year Question Papers Class 10 Maths 2013 Delhi Term 2
• CBSE Previous Year Question Papers Class 10 Maths 2012 Delhi Term 2
• CBSE Previous Year Question Papers Class 10 Maths 2011 Delhi Term 2
• CBSE Previous Year Question Papers Class 10 Maths 2016 Outside Delhi Term 2
• CBSE Previous Year Question Papers Class 10 Maths 2015 Outside Delhi Term 2
• CBSE Previous Year Question Papers Class 10 Maths 2014 Outside Delhi Term 2
• CBSE Previous Year Question Papers Class 10 Maths 2013 Outside Delhi Term 2
• CBSE Previous Year Question Papers Class 10 Maths 2012 Outside Delhi Term 2
• CBSE Previous Year Question Papers Class 10 Maths 2011 Outside Delhi Term 2

CBSE Previous Year Question Papers class 10 Maths

Last 10 Years Question Papers for Class 10 Maths are tabulated as follows:

CBSE Topper Answer Sheet Class 10 Maths

Download PDF of Last 5 Years Toppers Answer Sheet Photo Copies from the following table.

CBSE Class 10 Previous Year Question Paper Maths 2018

CBSE Class 10 Previous Year Question papers for Maths 2018 with marking scheme for main exam and compartment exam are tabulated as follows:

CBSE Previous Year Question Paper Class 10 Maths 2017

CBSE Class 10 Previous Year Question papers for Maths 2017 with marking scheme for main exam and compartment exam are tabulated as follows:

CBSE Previous Year Question Paper Class 10 Maths 2016

CBSE Class 10 Previous Year Question papers for Maths 2016 with marking scheme for main exam and compartment exam are tabulated as follows:

CBSE Previous Year Question Papers Class 10 Maths 2015

CBSE Class 10 Previous Year Question papers for Maths 2015 with marking scheme for main exam and compartment exam are tabulated as follows:

CBSE Previous Year Question Papers Class 10 Maths 2014

CBSE Class 10 Previous Year Question papers for Maths 2014 with marking scheme for main exam and compartment exam are tabulated as follows:

CBSE Sample Papers for Class 10 Maths SA2

CBSE Class 10 Sample Question papers for Maths 2017 SA2 are tabulated as follows:

Solved Papers For the Year 2017 :

• Solved CBSE Sample Paper for Class 10 SA2 Maths 2017 Set 1
• Solved CBSE Sample Paper for Class 10 SA2 Maths 2017 Set 2
• Solved CBSE Sample Paper for Class 10 SA2 Maths 2017 Set 3
• Solved CBSE Sample Paper for Class 10 SA2 Maths 2017 Set 4
• Solved CBSE Sample Paper for Class 10 SA2 Maths 2017  Set 5
• Solved CBSE Sample Paper for Class 10 SA2 Maths 2017 Set 6
• Solved CBSE Sample Paper for Class 10 SA2 Maths 2017 Set 7
• Solved CBSE Sample Paper for Class 10 SA2 Maths 2017 Set 8
• Solved CBSE Sample Paper for Class 10 SA2 Maths 2017 Set 9
• Solved CBSE Sample Paper for Class 10 SA2 Maths 2017 Set 10
• Solved CBSE Sample Paper for Class 10 SA2 Maths 2017 Set 11
• Solved CBSE Sample Paper for Class 10 SA2 Maths 2017 Set 12
• Solved CBSE Sample Paper for Class 10 SA2 Maths 2017 Set 13

Unsolved Papers:

• Sample paper for Maths Set A
• Sample paper for Maths Set B
• Sample paper for Maths Set C
• Sample paper for Maths Set D

More Resources for CBSE Class 10:

• RD Sharma Class 10 Solutions
• Lakhmir Singh Physics Class 10
• Solved Sample Papers Class 10 Science

Importance of Solving CBSE Class 10 Maths Sample Papers

1. By solving the CBSE Sample Papers for Class 10 Maths, students will get an idea of exam pattern, the types of questions asked and weightage of each section.
2. Solving CBSE Class 10 sample paper will be good practice for students.
3. These CBSE Maths Sample Papers covers all the important topics and also the questions which are repeated every year.
4. CBSE Class 10 Maths sample question paper will help the students to analyze their performance. This will also help with time management.
5. This will boost up the students’ confidence level.
6. After this much of practice, students will have a good idea about what to expect in exams.

We hope the CBSE Sample Questions papers for Class 10 Maths, help you. If you have any query regarding Maths Sample Questions papers for Class 10, drop a comment below and we will get back to you at the earliest.

The post CBSE Sample Papers for Class 10 Maths Standard and Basic with Solutions 2020-2021 appeared first on Learn CBSE.